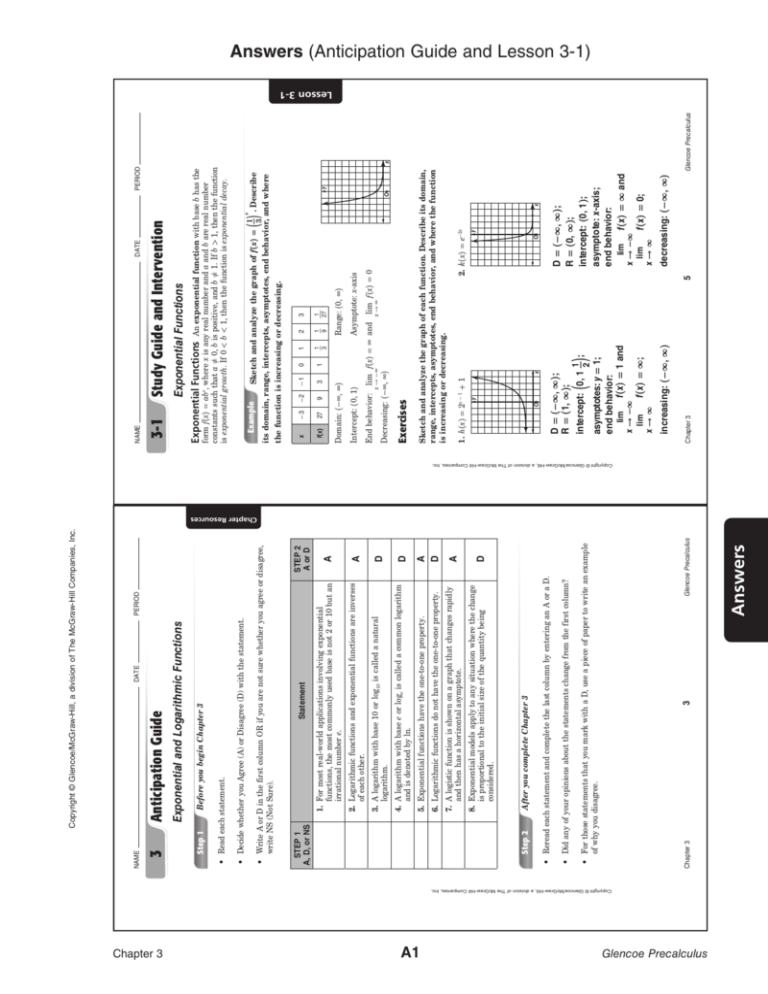
Chapter 3 Exponential And Logarithmic Functions Answer Key
Chapter 3 Exponential And Logarithmic Functions Answer Key - Is the population growing or shrinking? Web exponential functions grow exponentially—that is, very, very quickly. Web in this section, you will: 6.6 exponential and logarithmic equations; Video answers for all textbook questions of chapter 3, exponential and logarithmic functions, precalculus. In math, the logarithm is the inverse function to exponentiation. The material here is background material for the chapter on exponential and logarithmic functions and it is wise to review the sections on inverse functions prior to discussing logarithms. This function seems to “transcend” algebra. Four more steps, for example, bring the value to 2,048. X x f1x2 f1x2= 42.211.562x, objectives.
4.7 exponential and logarithmic models; 4.4 graphs of logarithmic functions; Logarithmic functions and exponential functions. Web chapter 3 exponential, logistic, and logarithmic functions section 3.1 exponential and logistic functions exploration 1 2. An asymptote is a line that the graph of a function approaches, as \(x\) either increases or decreases without bound. X x f1x2 f1x2= 42.211.562x, objectives. In math, the logarithm is the inverse function to exponentiation. By establishing the relationship between exponential and logarithmic functions, we can now solve basic logarithmic and exponential equations by rewriting. Web introduction to exponential and logarithmic functions; The point (0, 1) is common to all four graphs, and all four functions can.
Log7(49) = 2 can be written as an exponential equation as 72 = 49. Web in this section, you will: Logarithmic functions and exponential functions. 4.4 graphs of logarithmic functions; 4.2 graphs of exponential functions; Solve applied problems involving exponential and logarithmic. 4.6 exponential and logarithmic equations; 4.4 graphs of logarithmic functions; 6.2 graphs of exponential functions; In math, the logarithm is the inverse function to exponentiation.
PPT Chapter 8 Exponential and Logarithmic Functions PowerPoint
4.2 graphs of exponential functions; Web chapter 3, exponential and logarithmic functions video solutions, precalculus with limits | numerade. 4.6 exponential and logarithmic equations; 6.7 exponential and logarithmic models; Use logarithms to solve exponential equations.
PPT Chapter 7 Exponential and Logarithmic Functions PowerPoint
Web introduction to exponential and logarithmic functions; Logarithmic functions and their graphs. 4.2 graphs of exponential functions; An asymptote is a line that the graph of a function approaches, as \(x\) either increases or decreases without bound. 4.6 exponential and logarithmic equations;
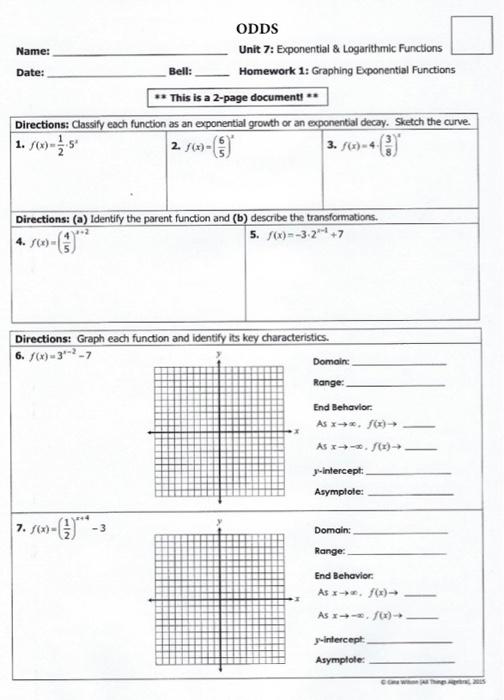
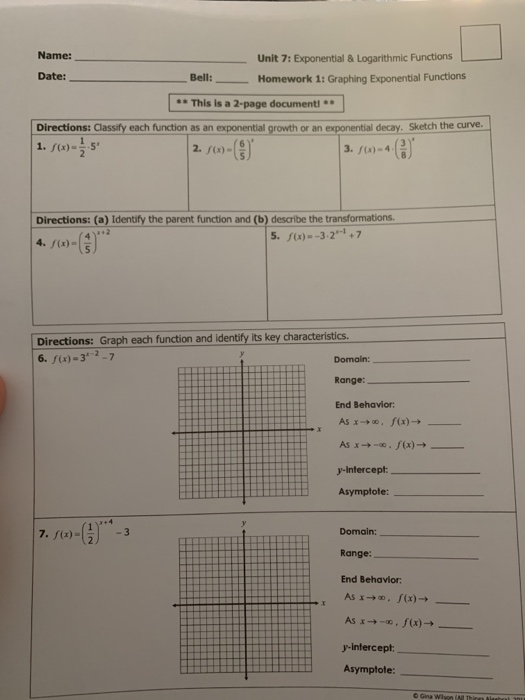
Solved ODDS Name Unit 7 Exponential & Logarithmic
Logarithmic functions and exponential functions. Web introduction to exponential and logarithmic functions; This function seems to “transcend” algebra. In this section we explore functions with a constant base and variable exponents. 6.2 graphs of exponential functions;
Algebra 2 Practice 8 5 Exponential And Logarithmic Equations Tessshebaylo
An asymptote is a line that the graph of a function approaches, as \(x\) either increases or decreases without bound. Use logarithms to solve exponential equations. The logarithm is actually the exponent to which the base is raised to obtain its argument. Logarithmic functions and their graphs. 4.4 graphs of logarithmic functions;
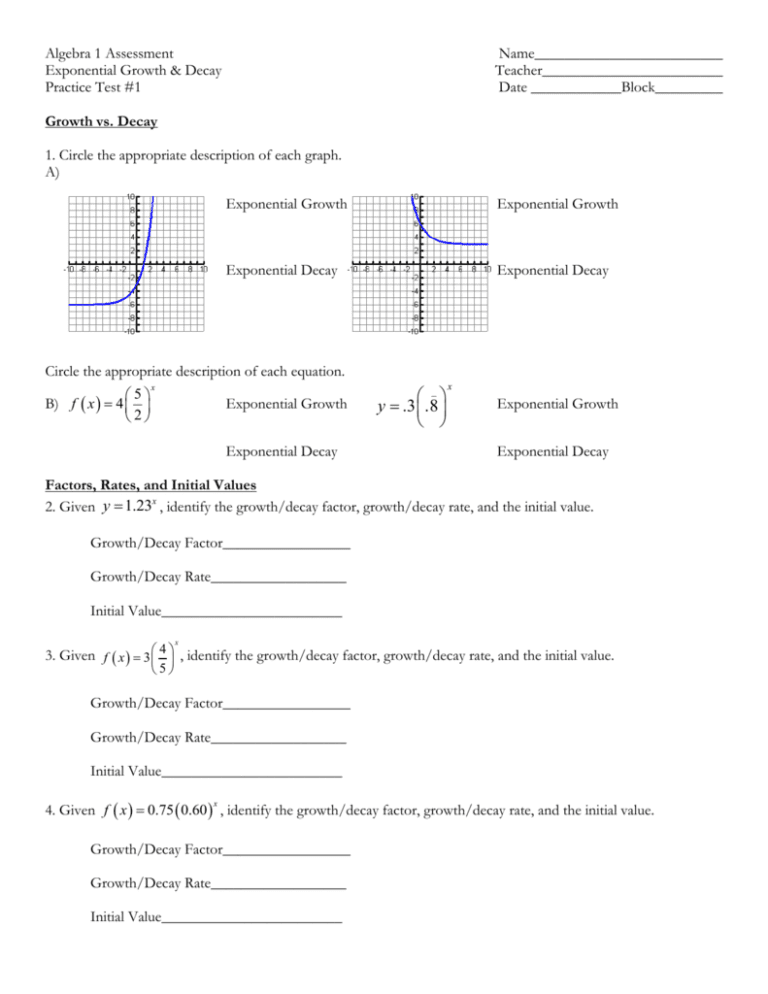
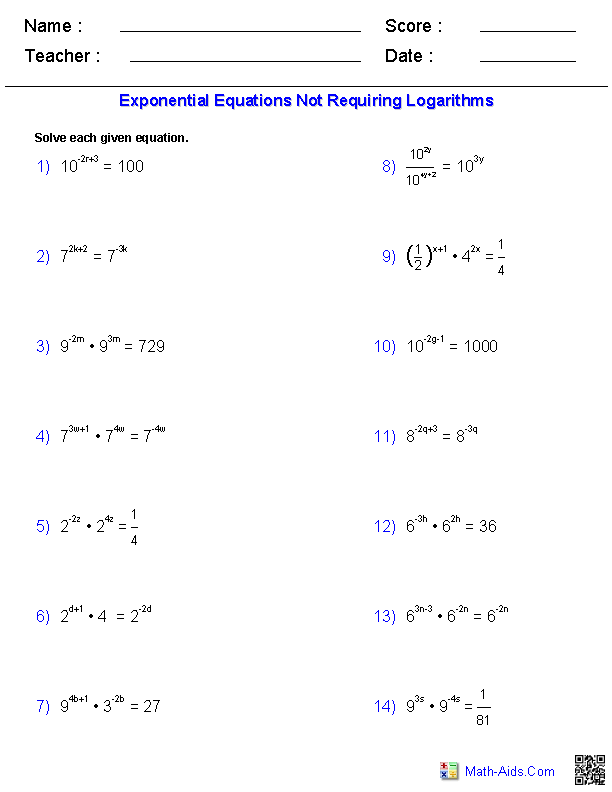
Exponential Functions Worksheet —
6.6 exponential and logarithmic equations; Step 4 estimate the gdp. The horizontal asymptote of an exponential function tells us the limit of the function… In math, the logarithm is the inverse function to exponentiation. Exponential functions and their graphs.
Chapter 7 Exponential And Logarithmic Functions Worksheet Answers
Web introduction to exponential and logarithmic functions; Four more steps, for example, bring the value to 2,048. Web chapter 3 exponential and logarithmic functions answer key. Log7(49) = 2 can be written as an exponential equation as 72 = 49. In math, the logarithm is the inverse function to exponentiation.
Chapter 3 Exponential and Logarithmic Functions
The point (0, 1) is common to all four graphs, and all four functions can. Logarithmic functions and exponential functions. 6.2 graphs of exponential functions; Web exponential functions grow exponentially—that is, very, very quickly. 4.7 exponential and logarithmic models;
Log And Exponential Equations Worksheet
In math, the logarithm is the inverse function to exponentiation. By establishing the relationship between exponential and logarithmic functions, we can now solve basic logarithmic and exponential equations by rewriting. Web introduction to exponential and logarithmic functions; Web this chapter studies functions, the associated notation, and key ideas necessary for analyzing graphs in calculus. Web f(x) = 2x y =.
Algebra 2 Graphing Linear Inequalities Practice Answer Key 6th Grade
Product and quotient properties of logarithms; 4.4 graphs of logarithmic functions; Web this chapter studies functions, the associated notation, and key ideas necessary for analyzing graphs in calculus. An asymptote is a line that the graph of a function approaches, as \(x\) either increases or decreases without bound. Web chapter 3, exponential and logarithmic functions video solutions, precalculus with limits.
1 3 Skills Practice Solving Equations Glencoe Algebra 2 Tessshebaylo
In this section we explore functions with a constant base and variable exponents. 6.7 exponential and logarithmic models; Use the definition of a logarithm to solve logarithmic equations. 6.4 graphs of logarithmic functions; Web chapter 3 exponential, logistic, and logarithmic functions section 3.1 exponential and logistic functions exploration 1 2.
Log3(81) = 4 Can Be Written As An Exponential Equation As 34 = 81.
Web in this section, you will: Use the definition of a logarithm to solve logarithmic equations. Logarithmic functions and their graphs. Web introduction to exponential and logarithmic functions;
The Logarithm Is Actually The Exponent To Which The Base Is Raised To Obtain Its Argument.
4.4 graphs of logarithmic functions; Video answers for all textbook questions of chapter 3, exponential and logarithmic functions, precalculus. Web this chapter studies functions, the associated notation, and key ideas necessary for analyzing graphs in calculus. The material here is background material for the chapter on exponential and logarithmic functions and it is wise to review the sections on inverse functions prior to discussing logarithms.
6.7 Exponential And Logarithmic Models;
A logarithmic equation is a calculation that involves the logarithm of an expression containing a variable. By establishing the relationship between exponential and logarithmic functions, we can now solve basic logarithmic and exponential equations by rewriting. The point (0, 1) is common to all four graphs, and all four functions can. 4.6 exponential and logarithmic equations;
Is The Population Growing Or Shrinking?
4.7 exponential and logarithmic models; In this section we explore functions with a constant base and variable exponents. Web f(x) = 2x y = 2x ⇒ x = 2y we quickly realize that there is no method for solving for y. 4.6 exponential and logarithmic equations;