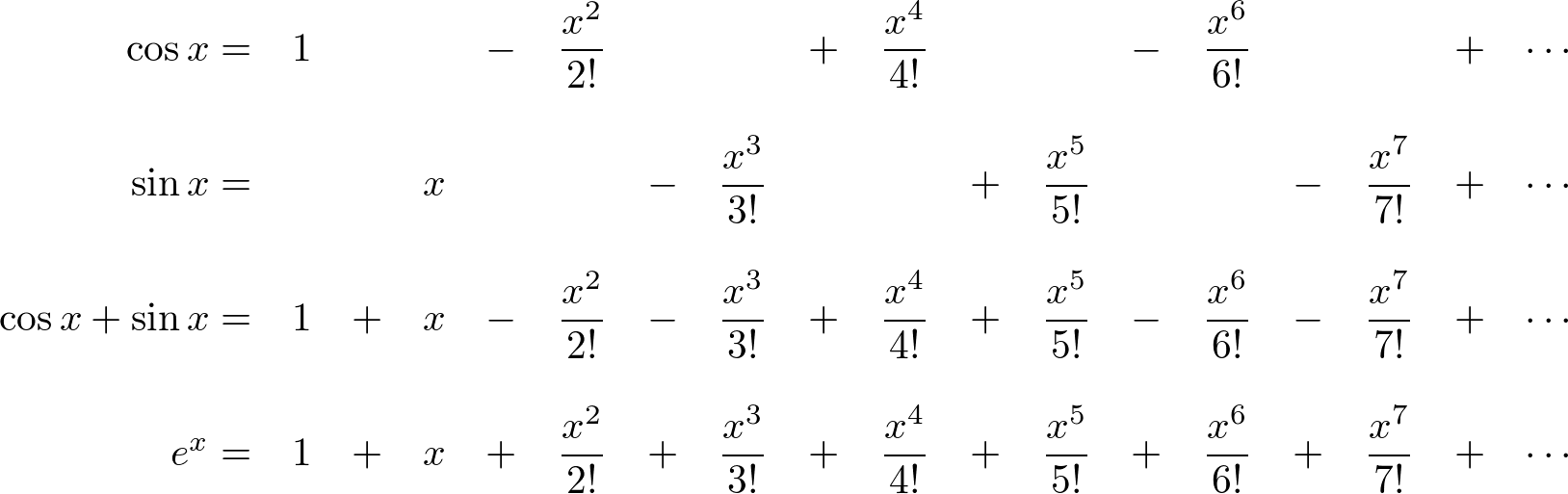Cos X In Exponential Form
Cos X In Exponential Form - Web calculate exp × the function exp calculates online the exponential of a number. This formula can be interpreted as saying that the function e is a unit complex number, i.e., it traces out the unit circle in the complex plane as φ ranges through the real numbers. We can now use this complex exponential. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: Converting complex numbers from polar to exponential form. Eit = cos t + i. Andromeda on 7 nov 2021. Here φ is the angle that a line connecting the origin with a point on the unit circle makes with the positive real axis, measured counterclockwise and in radians. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all.
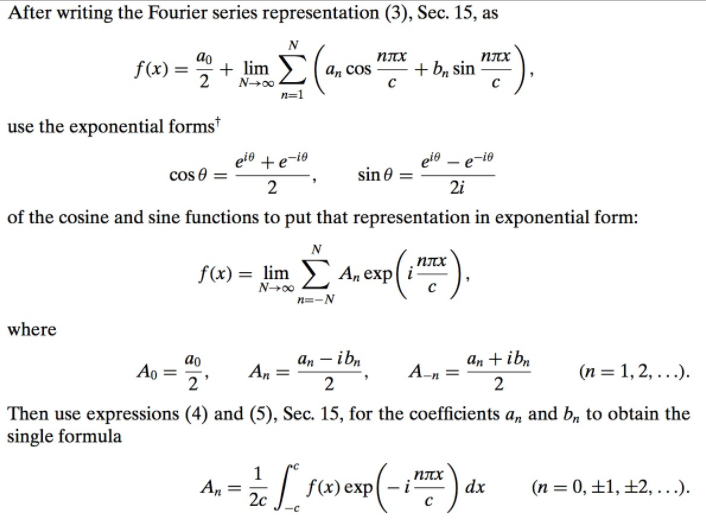
Web i am in the process of doing a physics problem with a differential equation that has the form: Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: The odd part of the exponential function, that is, sinh x = e x − e − x 2 = e 2 x − 1 2 e x = 1 − e − 2 x 2 e − x. We can now use this complex exponential. Web an exponential equation is an equation that contains an exponential expression of the form b^x, where b is a constant (called the base) and x is a variable. Web answer (1 of 10): Y = acos(kx) + bsin(kx) according to my notes, this can also be. Andromeda on 7 nov 2021. Web calculate exp × the function exp calculates online the exponential of a number. Web complex exponential series for f(x) defined on [ − l, l].
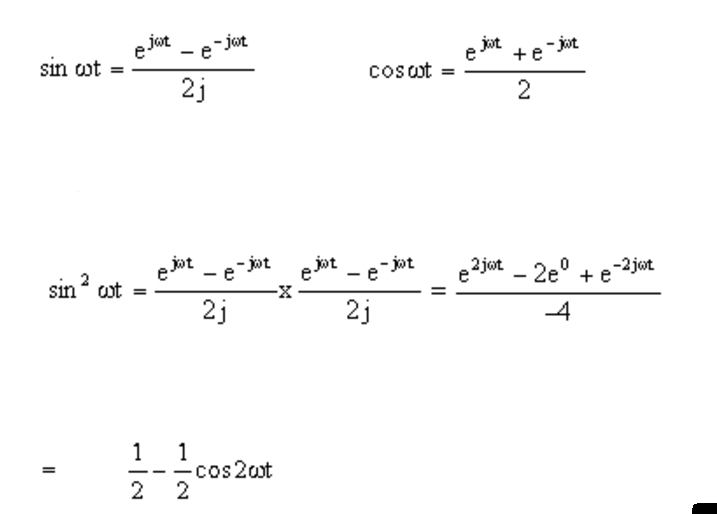
Here φ is the angle that a line connecting the origin with a point on the unit circle makes with the positive real axis, measured counterclockwise and in radians. Andromeda on 7 nov 2021. We can now use this complex exponential. Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: Put 𝑍 equals four times the square. Y = acos(kx) + bsin(kx) according to my notes, this can also be. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as real $x$. Put 𝑍 = (4√3) (cos ( (5𝜋)/6) − 𝑖 sin (5𝜋)/6) in exponential form. Eit = cos t + i.
Other Math Archive January 29, 2018
Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: Y = acos(kx) + bsin(kx) according to my notes, this can also be. Web i am in the process of doing a physics problem with a differential equation that has the form: We can now.
Example 23 Differentiate sin (cos (x^2)) Teachoo Examples
The odd part of the exponential function, that is, sinh x = e x − e − x 2 = e 2 x − 1 2 e x = 1 − e − 2 x 2 e − x. Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as.
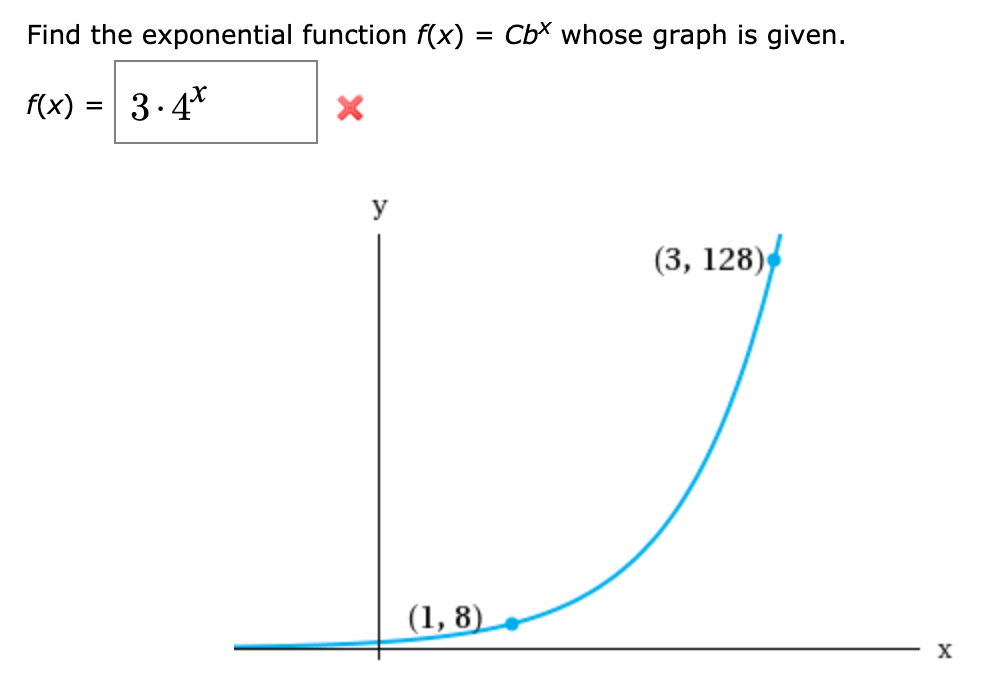
Solved A) Find The Exponential Function Whose Graph Is Gi...
Put 𝑍 equals four times the square. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web i am in the process of doing a physics problem with a differential equation that has the form: This formula can be interpreted as saying that the function e is.
PPT Chapter 7 Fourier Series PowerPoint Presentation, free download
Eit = cos t + i. The odd part of the exponential function, that is, sinh x = e x − e − x 2 = e 2 x − 1 2 e x = 1 − e − 2 x 2 e − x. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn =.
C Practical and Assignment Programscos(x) YouTube
Web answer (1 of 10): F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. The odd part of the exponential function, that is, sinh x = e x − e − x 2 = e 2 x − 1 2 e x = 1 − e − 2.
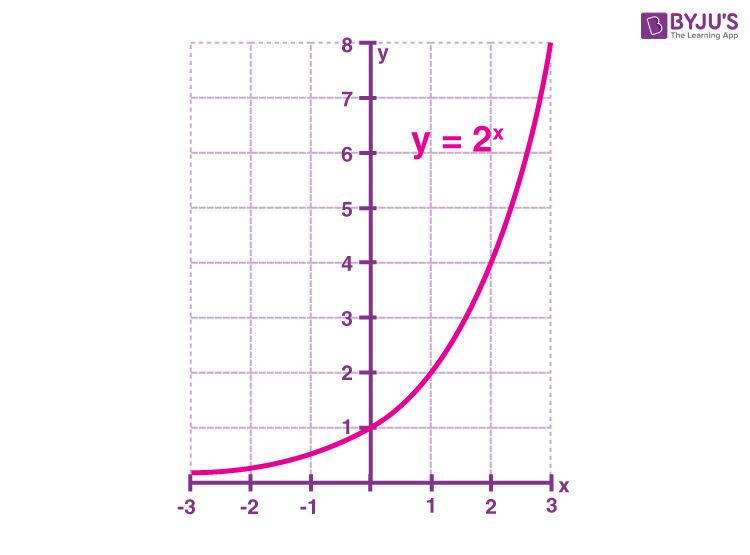
Exponential Functions Definition, Formula, Properties, Rules
Andromeda on 7 nov 2021. Converting complex numbers from polar to exponential form. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Put 𝑍 equals four times the square. The odd part of the exponential function, that is, sinh x = e x − e −.
express cos x as exponential YouTube
Web calculate exp × the function exp calculates online the exponential of a number. Eit = cos t + i. Web answer (1 of 10): Web relations between cosine, sine and exponential functions. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all.
Euler's Equation
Converting complex numbers from polar to exponential form. Web an exponential equation is an equation that contains an exponential expression of the form b^x, where b is a constant (called the base) and x is a variable. Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as real $x$. Here.
Basics of QPSK modulation and display of QPSK signals Electrical
This formula can be interpreted as saying that the function e is a unit complex number, i.e., it traces out the unit circle in the complex plane as φ ranges through the real numbers. We can now use this complex exponential. Converting complex numbers from polar to exponential form. Web complex exponential series for f(x) defined on [ − l,.
Exponential growth Wikipedia
Eit = cos t + i. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as real $x$. Put 𝑍 equals four times the square. This formula can be interpreted.
Web $$E^{Ix} = \Cos X + I \Sin X$$ Fwiw, That Formula Is Valid For Complex $X$ As Well As Real $X$.
Web complex exponential series for f(x) defined on [ − l, l]. Y = acos(kx) + bsin(kx) according to my notes, this can also be. Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: The odd part of the exponential function, that is, sinh x = e x − e − x 2 = e 2 x − 1 2 e x = 1 − e − 2 x 2 e − x.
We Can Now Use This Complex Exponential.
This formula can be interpreted as saying that the function e is a unit complex number, i.e., it traces out the unit circle in the complex plane as φ ranges through the real numbers. Andromeda on 7 nov 2021. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Here φ is the angle that a line connecting the origin with a point on the unit circle makes with the positive real axis, measured counterclockwise and in radians.
Web An Exponential Equation Is An Equation That Contains An Exponential Expression Of The Form B^x, Where B Is A Constant (Called The Base) And X Is A Variable.
Web relations between cosine, sine and exponential functions. Put 𝑍 equals four times the square. Eit = cos t + i. Converting complex numbers from polar to exponential form.
Put 𝑍 = (4√3) (Cos ( (5𝜋)/6) − 𝑖 Sin (5𝜋)/6) In Exponential Form.
E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web answer (1 of 10): F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web i am in the process of doing a physics problem with a differential equation that has the form: