Implicit Form Differential Equation
Implicit Form Differential Equation - To perform implicit differentiation on an equation that defines a function \(y\) implicitly in terms of a variable \(x\), use the. Questions tips & thanks want to join the conversation? Web with implicit differentiation, you're transforming expressions. This is the formula for a circle with a centre at (0,0) and. This is done using the chain rule, and viewing y as an implicit function of x. For example, according to the. For example, the implicit equation of the unit. In applications, the functions generally represent physical. Web the implicit solution of this differential equation is $x^2+y(x)^2=r^2$; Web implicit differentiation is a way of differentiating when you have a function in terms of both x and y.
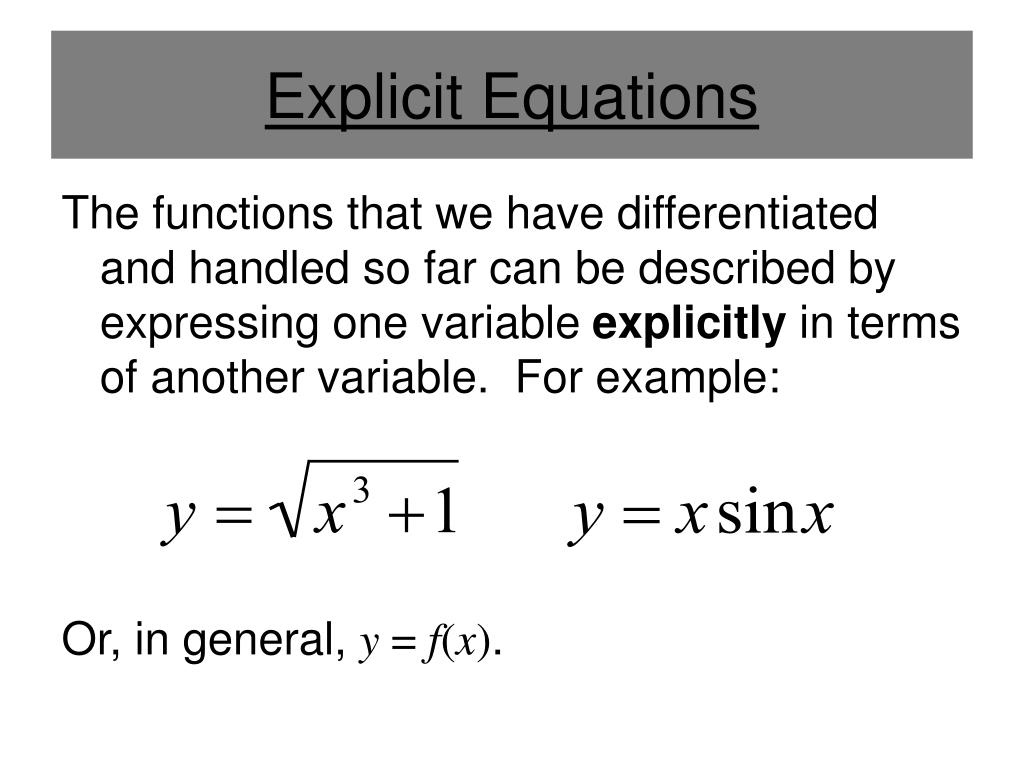
In most discussions of math, if the dependent variable y is a function of the independent variable x, we express y in terms of x. There is one differential equation that. Yet sometimes you just can't come up with a neat y. There are two ways to define functions, implicitly and explicitly. This is done using the chain rule, and viewing y as an implicit function of x. Web with implicit differentiation, you're transforming expressions. In applications, the functions generally represent physical. If this is the case, we say that y. Web a differential equation is any equation which contains derivatives, either ordinary derivatives or partial derivatives. Web in mathematics, an implicit equation is a relation of the form where r is a function of several variables (often a polynomial ).
Web with implicit differentiation, you're transforming expressions. For example, the implicit equation of the unit. Web a differential equation is any equation which contains derivatives, either ordinary derivatives or partial derivatives. Yet sometimes you just can't come up with a neat y. There is one differential equation that. Web the implicit solution of this differential equation is $x^2+y(x)^2=r^2$; Unfortunately, not all the functions. Web implicit differentiation helps us find dy/dx even for relationships like that. D/dx becomes an algebraic operation like sin or square root, and can perform it on both sides of an equation. Separating differential equations into x and y parts is fine;
Calculus Implicit Differentiation YouTube
There is one differential equation that. Questions tips & thanks want to join the conversation? This is done using the chain rule, and viewing y as an implicit function of x. Web to this point we’ve done quite a few derivatives, but they have all been derivatives of functions of the form y = f (x) y = f (.
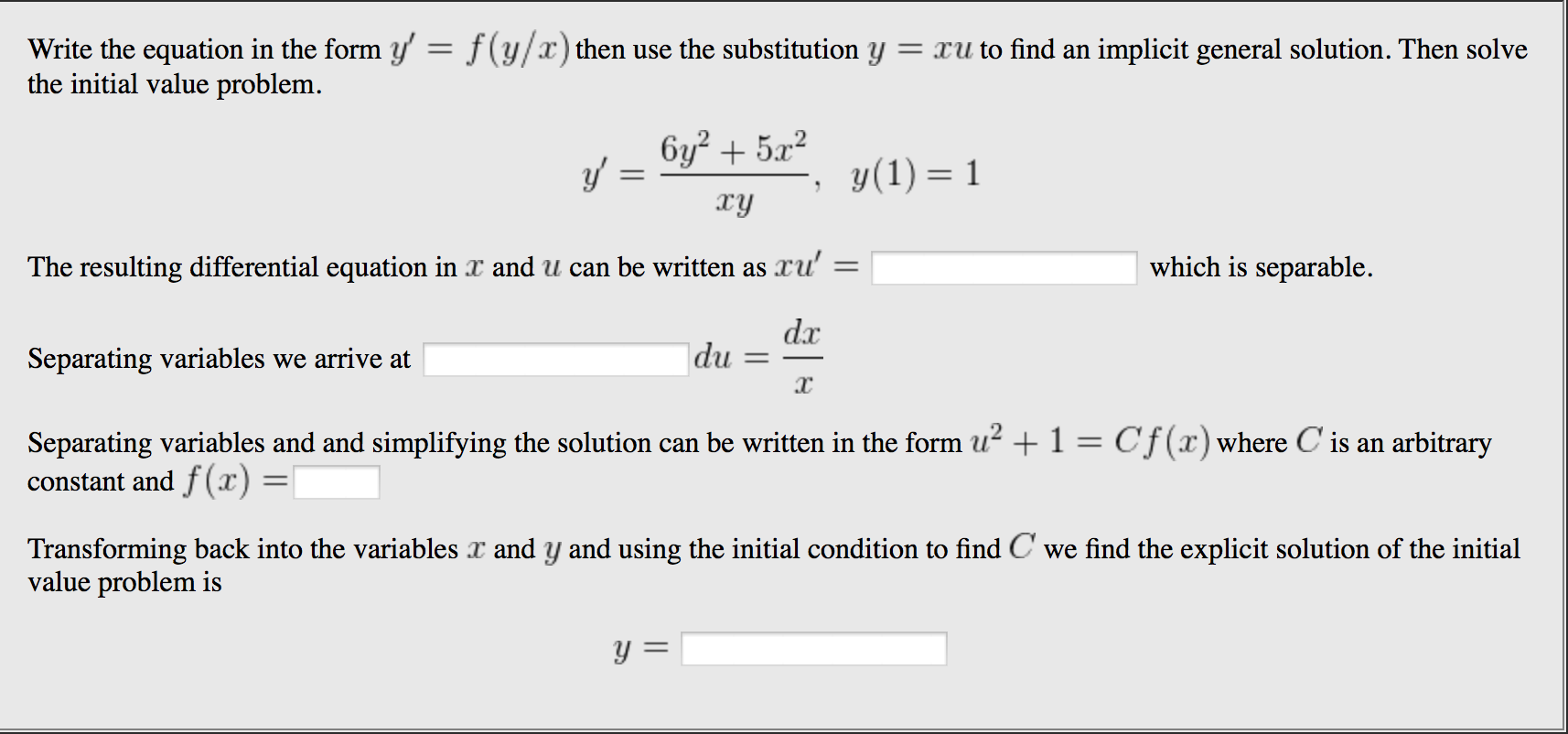
Solved Write The Equation In The Form Then Use The Substi...
This is done using the chain rule, and viewing y as an implicit function of x. Web the implicit solution of this differential equation is $x^2+y(x)^2=r^2$; There is one differential equation that. Web a differential equation is any equation which contains derivatives, either ordinary derivatives or partial derivatives. Yet sometimes you just can't come up with a neat y.
Differential Equations Explicit Solution YouTube
To perform implicit differentiation on an equation that defines a function \(y\) implicitly in terms of a variable \(x\), use the. If this is the case, we say that y. For example, according to the. Separating differential equations into x and y parts is fine; This is the formula for a circle with a centre at (0,0) and.
Core 4 Implicit Differentiation 1
Here $y(x)$ is implicitly defined. Unfortunately, not all the functions. If this is the case, we say that y. Web implicit differential equation of type \(y = f\left( {x,y'} \right).\) here we consider a similar case, when the variable \(y\) is an explicit function of \(x\) and \(y'.\) introduce the. Web up to 5% cash back finding implicit solutions.
PPT Implicit Differentiation PowerPoint Presentation, free download
There are two ways to define functions, implicitly and explicitly. Web up to 5% cash back finding implicit solutions. In applications, the functions generally represent physical. Web to find the implicit derivative, take the derivative of both sides of the equation with respect to the independent variable then solve for the derivative of the dependent variable with. Web a differential.
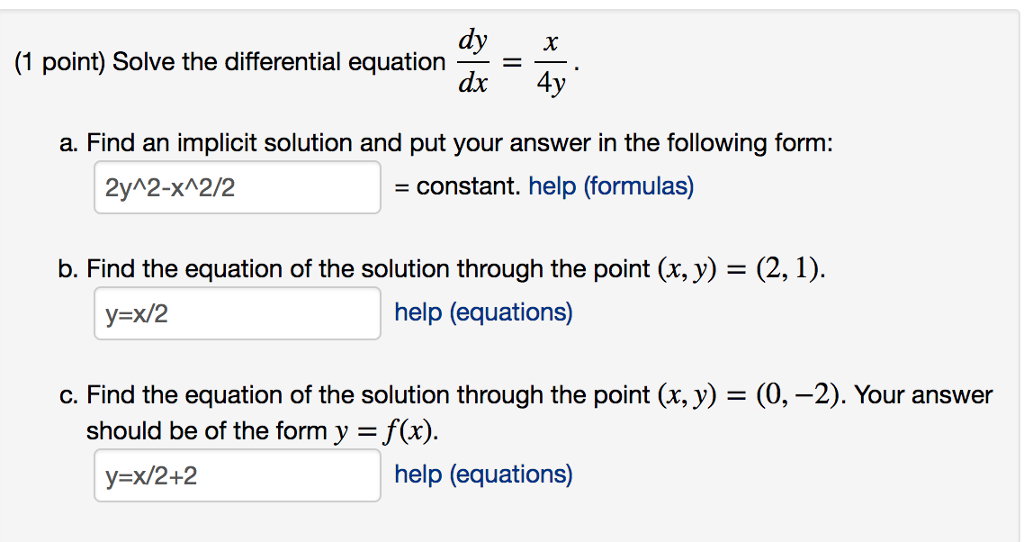
Solved (1 point) Solve the differential equation
Yet sometimes you just can't come up with a neat y. Web a differential equation is any equation which contains derivatives, either ordinary derivatives or partial derivatives. Unfortunately, not all the functions. This is done using the chain rule, and viewing y as an implicit function of x. Web given an implicit equation in x and y, finding the expression.
PPT Section 2.5 Implicit Differentiation PowerPoint Presentation
There are two ways to define functions, implicitly and explicitly. The graph of $$8x^3e^{y^2} = 3$$ is shown below. Web in mathematics, a differential equation is an equation that relates one or more unknown functions and their derivatives. Web given an implicit equation in x and y, finding the expression for the second derivative of y with respect to x..
Differential Equations (Part 2 Implicit Differentiation) YouTube
Web the implicit solution of this differential equation is $x^2+y(x)^2=r^2$; Web in mathematics, an implicit equation is a relation of the form where r is a function of several variables (often a polynomial ). Here $y(x)$ is implicitly defined. Web in mathematics, a differential equation is an equation that relates one or more unknown functions and their derivatives. In applications,.
3.2 implicit equations and implicit differentiation
Web the implicit solution of this differential equation is $x^2+y(x)^2=r^2$; There is one differential equation that. Questions tips & thanks want to join the conversation? For example, according to the. Unfortunately, not all the functions.
How to solve implicit differential equation? Mathematics Stack Exchange
Web in mathematics, a differential equation is an equation that relates one or more unknown functions and their derivatives. For example, according to the. Web to find the implicit derivative, take the derivative of both sides of the equation with respect to the independent variable then solve for the derivative of the dependent variable with. Web implicit differentiation is a.
Web Answer (1 Of 3):
The graph of $$8x^3e^{y^2} = 3$$ is shown below. If this is the case, we say that y. To perform implicit differentiation on an equation that defines a function \(y\) implicitly in terms of a variable \(x\), use the. The other answer has more detail — but to put it more simply, an explicit solution gives us our dependent variable as a function of our independent variable.
Web Up To 5% Cash Back Finding Implicit Solutions.
Here $y(x)$ is implicitly defined. Web in mathematics, a differential equation is an equation that relates one or more unknown functions and their derivatives. This is the formula for a circle with a centre at (0,0) and. Web the implicit solution of this differential equation is $x^2+y(x)^2=r^2$;
In Most Discussions Of Math, If The Dependent Variable Y Is A Function Of The Independent Variable X, We Express Y In Terms Of X.
It can also be quite helpful. D/dx becomes an algebraic operation like sin or square root, and can perform it on both sides of an equation. Separating differential equations into x and y parts is fine; Web to find the implicit derivative, take the derivative of both sides of the equation with respect to the independent variable then solve for the derivative of the dependent variable with.
Unfortunately, Not All The Functions.
For example, according to the. Yet sometimes you just can't come up with a neat y. In applications, the functions generally represent physical. Web to this point we’ve done quite a few derivatives, but they have all been derivatives of functions of the form y = f (x) y = f ( x).