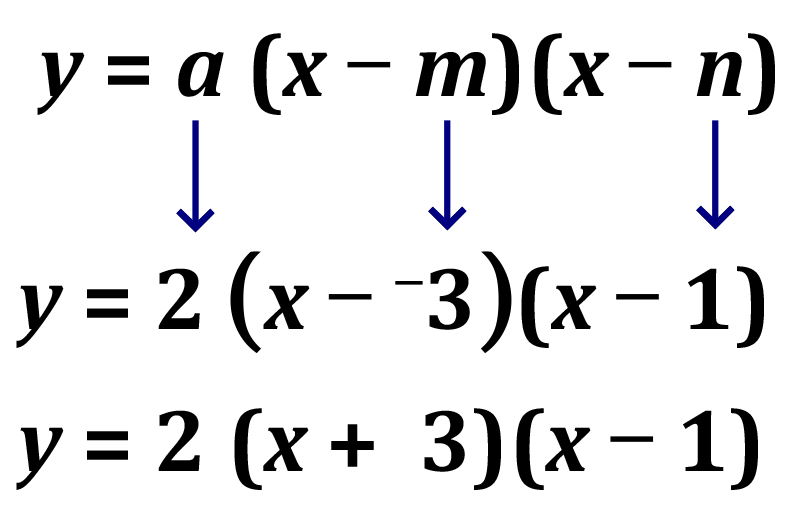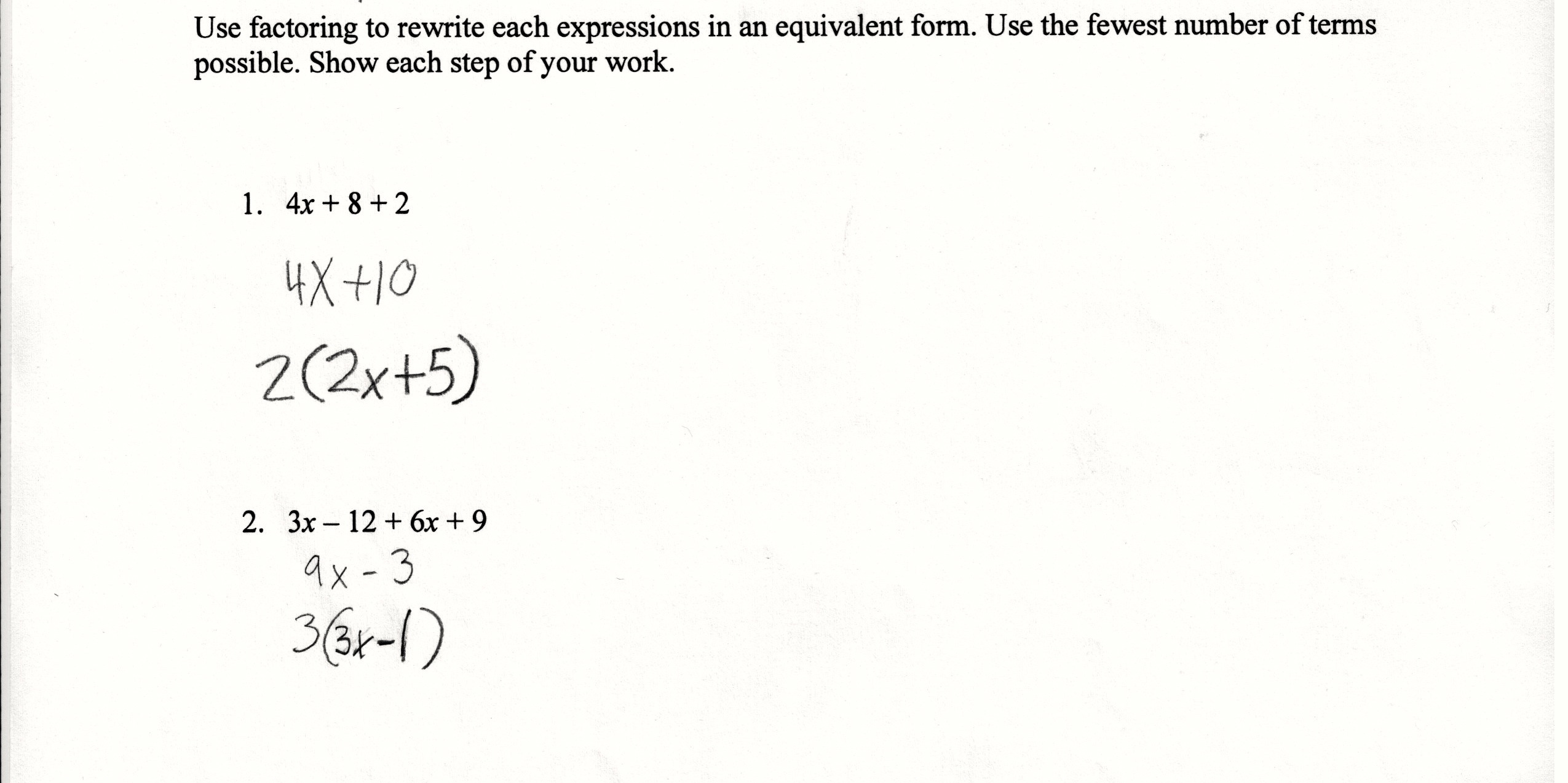What Is The Factored Form Of N 2 N

What Is The Factored Form Of N 2 N - N!, there are other ways of expressing it. N ⋅ (n −1)(n − 2)(n − 3)! Web factorial (n!) the factorial of n is denoted by n! = (n +2)(n + 1)n! To factor a trinomial x^2+bx+c find two. Trying to factor by splitting the middle term. = (n +2)(n + 1)(n)(n −1).1. Find a pair of integers whose product is c and whose sum is b. While there isn't a simplification of (2n)! Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer a a and k k.

= (n +2)(n + 1)n! N!, there are other ways of expressing it. Since both terms are perfect squares, factor using the difference of squares formula, a2 −b2 =. Rewrite 25 25 as 52 5 2. Web factor (n − (− 2 − 1)) (n − ( 2 − 1)) steps using the quadratic formula steps using direct factoring method view solution steps evaluate n2 + 2n − 1 quiz polynomial n2 +2n−1. = (n +2)(n + 1)(n)(n −1).1. Web answer (1 of 4): Trying to factor by splitting the middle term. (n − 2) (n −. Web n^2 + n.

In this case, whose product is. Web to factor a binomial, write it as the sum or difference of two squares or as the difference of two cubes. Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer a a and k k. N2 − n − 72. To find a and b, set up a system. = (n + 2)(n + 1)n! Let f(x) = f ( x) = numbers of positive divisors of the integer x x. To factor a trinomial x^2+bx+c find two. = where you used the fact that n! N ⋅ (n −1)(n − 2) (n − 3)!

Learn It Click to Reveal

N ⋅ (n −1)(n − 2) (n − 3)! Trying to factor by splitting the middle term. Web this is a very interesting question. N!, there are other ways of expressing it. In this case, whose product is.

How to write a quadratic function in factored form to represent a

= (n +2)(n + 1)(n)(n −1).1. Web this is a very interesting question. Web the factored form of a quadratic equation \(ax^2 +bx+c=0 \) can be obtained by various methods. In this case, whose product is. Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer a a and k k.

2) Factored/Intercept Form

= (n +2)(n + 1)n! Web to factor a binomial, write it as the sum or difference of two squares or as the difference of two cubes. = n−1 ∏ k=0(2n −k) = (2n)(2n − 1).(n +1) this. N ⋅ (n −1)(n − 2) (n − 3)! N ⋅ (n −1)(n − 2)(n − 3)!

intro to factored form YouTube

Find a pair of integers whose product is c c and whose sum. Web answer (1 of 4): To find a and b, set up a system. You will see that n^2/n =n. And calculated by the product of integer numbers from 1 to n.

3.5 Graphing with factored form YouTube

Depending upon the case, a suitable method is applied to find the factors. = n−1 ∏ k=0(2n −k) = (2n)(2n − 1).(n +1) this. N ⋅ (n −1)(n − 2)(n − 3)! Therefore n (n+1) arrow right. Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer a a and k k.

SOLVEDWrite in factored form by factoring out th…

Web answer (1 of 4): Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer a a and k k. = (n + 2)(n + 1)n! Therefore n (n+1) arrow right. Find a pair of integers whose product is c c and whose sum.

Factored Form what does it tell us?! YouTube

= (n + 2)(n + 1)n! Find a pair of integers whose product is c c and whose sum. You will see that n^2/n =n. N ⋅ (n −1)(n − 2) (n − 3)! = n−1 ∏ k=0(2n −k) = (2n)(2n − 1).(n +1) this.

Factored Forms

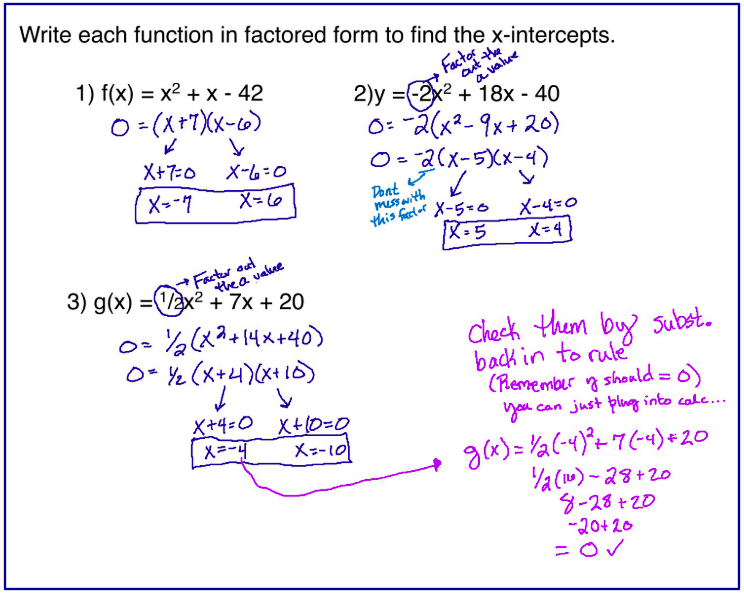
While there isn't a simplification of (2n)! Consider the form x2 + bx+c x 2 + b x + c. Find a pair of integers whose product is c c and whose sum. How do you factor a trinomial? Web the factored form of a quadratic equation \(ax^2 +bx+c=0 \) can be obtained by various methods.

Factored Form

Therefore n (n+1) arrow right. Find a pair of integers whose product is c c and whose sum. Since both terms are perfect squares, factor using the difference of squares formula, a2 −b2 =. To factor a trinomial x^2+bx+c find two. Web factor (n − (− 2 − 1)) (n − ( 2 − 1)) steps using the quadratic formula.

PPT Factored Form PowerPoint Presentation, free download ID4119177

Consider the form x2 + bx+c x 2 + b x + c. Web the factored form of a quadratic equation \(ax^2 +bx+c=0 \) can be obtained by various methods. Depending upon the case, a suitable method is applied to find the factors. Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer.

Trying To Factor By Splitting The Middle Term.

Web n^2 + n. Web this is a very interesting question. Rewrite 25 25 as 52 5 2. If you divide the whole thing by n.

To Factor A Trinomial X^2+Bx+C Find Two.

= n−1 ∏ k=0(2n −k) = (2n)(2n − 1).(n +1) this. (n − 2) (n −. = where you used the fact that n! Web the factored form of a quadratic equation \(ax^2 +bx+c=0 \) can be obtained by various methods.

We Can Write It As:

= (n +2)(n + 1)(n)(n −1).1. N!, there are other ways of expressing it. And calculated by the product of integer numbers from 1 to n. To find a and b, set up a system.